Is the universe bounded or unbounded?

[otseng]

In the Light, stars, and creationism thread, I proposed a theory to reconcile a young earth with being able to see stars that are billions of light years away. The theory assumes that the Big Bang is true, however, it also assumes that the universe is bounded. In typical cosmology, it is assumed that the universe is unbounded.

Bounded means that the universe has a boundary to it. There exists an "edge" to the universe in which beyond this boundary, our universe does not exist.

In an unbounded universe, there is no "edge". The universe "wraps" around itself. So, if you are to go in any direction in a straight line, you will eventually come back to the starting point.

This is hard to conceptualize, but can be explained like a surface of a sphere. On the surface of a sphere, if you start at any point and then go in a straight line, you will eventually come back to the starting point. Now, instead a 2-D surface on a sphere, the universe is a 3-D topology that curves in on itself.

The ramifications of either of these two assumptions make for drastically different cosmological conclusions.

So, the questions are:
1. Is the universe bounded or unbounded? Why?
2. What are the ramifications of whether it is bounded or unbounded?

[otseng]

At any point inside a homogeneous sphere the gravity only depends on the mass within a sub-sphere whose radius ends at that point. All mass beyond this radius can be considered as collection of spherical shells as per the limiting case of the hollow sphere, and hence contributes nothing to the field inside the radius.

Still not quite clear on your meaning here.

Here is my attempt at a visual representation:

The blue represents the solid universe. The red dot represents the observer. The yellow represents the circle in which we can consider the gravity to be cancelled out. So, the part that's left (blue) would be what would have an effect on the red dot.

Because the extremes of red-shift seen in distant galaxies can only be due to cosmological expansion.

I'm suspecting a bit of circular logic here. Red-shifts explains metric expansion. And metric expansion explains red-shifts.

The "cosmic speed limiter" prevents lines of distance/time exceeding 45 degrees on the diagram as that angle has been chosen to represent light-speed. So for any point we might place ourselves at, such as the one at top center, then looking out and back in time, information beyond the (red) line at 45 deg, to the normal cannot reach us.

OK, I think I get it now. The observer would only be at the top point where the two red lines meet at 90 degrees.

[QED]

Still not quite clear on your meaning here.

Here is my attempt at a visual representation:

The blue represents the solid universe. The red dot represents the observer. The yellow represents the circle in which we can consider the gravity to be cancelled out. So, the part that's left (blue) would be what would have an effect on the red dot.

Diagrams! What a good idea

Here's my original description again with a diagram: In an idealized model, Gravity cancels out at all points inside a hollow sphere: (depicted along the top row) At the exact center of a sphere, there will obviously be an equal gravitational effect from every point on the outer shell of the sphere. Moving from the center to some other point inside the sphere, as the point approaches one edge, the forces from that side become stronger than the other. However, there is a compensating change taking place as the amount of mass behind the point gets larger, and this cancels out exactly as described by Gauss's law (thus there is no net field at all on the top row).





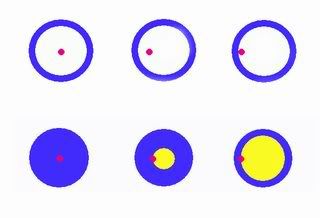
(moving to the bottom row) At any point inside a homogeneous sphere the gravity only depends on the mass within a sub-sphere (yellow) whose radius ends at that point. All mass beyond this radius can be considered as collection of spherical shells as per the limiting case of the hollow sphere, and hence contributes nothing to the (yellow) field inside the radius.

Because the extremes of red-shift seen in distant galaxies can only be due to cosmological expansion.

I'm suspecting a bit of circular logic here. Red-shifts explains metric expansion. And metric expansion explains red-shifts.

Saying that red shift can only be due to cosmological (metric) expansion, we are only admitting that we cannot accept superluminal velocities in a fixed metric, and that we know of no other practical mechanism that would create the appearance of Doppler.

[otseng]

Here's my original description again with a diagram: In an idealized model, Gravity cancels out at all points inside a hollow sphere: (depicted along the top row) At the exact center of a sphere, there will obviously be an equal gravitational effect from every point on the outer shell of the sphere. Moving from the center to some other point inside the sphere, as the point approaches one edge, the forces from that side become stronger than the other. However, there is a compensating change taking place as the amount of mass behind the point gets larger, and this cancels out exactly as described by Gauss's law (thus there is no net field at all on the top row).



(moving to the bottom row) At any point inside a homogeneous sphere the gravity only depends on the mass within a sub-sphere (yellow) whose radius ends at that point. All mass beyond this radius can be considered as collection of spherical shells as per the limiting case of the hollow sphere, and hence contributes nothing to the (yellow) field inside the radius.

Let's just look at the top figures for now.

If the object is at the exact center, I would say the width of the blue line would be zero. The white area that would represent the cancelled out gravitational force would extend to the edge.

Why would the white area (cancelled out gravitational force) remain the same if the object moved to the left? Wouldn't the cancelled out gravitational force be represented by a circle where the object is at the center? (Like my diagrams above) The force acting upon the object should change as the object moves.

Saying that red shift can only be due to cosmological (metric) expansion, we are only admitting that we cannot accept superluminal velocities in a fixed metric, and that we know of no other practical mechanism that would create the appearance of Doppler.

Gravitational red shifting could also be another mechanism.

[QED]

Here's my original description again with a diagram: In an idealized model, Gravity cancels out at all points inside a hollow sphere: (depicted along the top row) At the exact center of a sphere, there will obviously be an equal gravitational effect from every point on the outer shell of the sphere. Moving from the center to some other point inside the sphere, as the point approaches one edge, the forces from that side become stronger than the other. However, there is a compensating change taking place as the amount of mass behind the point gets larger, and this cancels out exactly as described by Gauss's law (thus there is no net field at all on the top row).



(moving to the bottom row) At any point inside a homogeneous sphere the gravity only depends on the mass within a sub-sphere (yellow) whose radius ends at that point. All mass beyond this radius can be considered as collection of spherical shells as per the limiting case of the hollow sphere, and hence contributes nothing to the (yellow) field inside the radius.

Let's just look at the top figures for now.

If the object is at the exact center, I would say the width of the blue line would be zero. The white area that would represent the cancelled out gravitational force would extend to the edge.

Why would the white area (cancelled out gravitational force) remain the same if the object moved to the left? Wouldn't the cancelled out gravitational force be represented by a circle where the object is at the center? (Like my diagrams above) The force acting upon the object should change as the object moves.

No, in all three diagrams on the top row, the point denoted by the red dot experiences the same field in each position. The blue shell is mass, the white is void. If the dot moves closer to the shell the field (at the point) remains in balance because the greater part of the mass of the shell comes into "view" behind it (albeit at a distance). Maybe you're not seeing this because you're not familiar with the "magic" of Gauss's law which keeps the competing inverse-square law effects perfectly in balance. It's not meant to be an accurate depiction of what's going on, but this picture should help:



What I'm trying to show is that lots of weak vectors balance fewer strong ones. The sum of all vectors to the right of the point is always equal to those on the left (the same would apply if we also moved the point up or down). If I had more time I'd put signed numbers on the vectors and show them summing to zero, but you can probably imagine it for me

Saying that red shift can only be due to cosmological (metric) expansion, we are only admitting that we cannot accept superluminal velocities in a fixed metric, and that we know of no other practical mechanism that would create the appearance of Doppler.

Gravitational red shifting could also be another mechanism.

That's why we've switched to discussing gravitational fields. I've been trying to explain why your gravitational model for the universe is wrong! Going back to our table of different kinds of redshift

Gravitational red shifts are generally very small, and you only get very large ones from the light emitted near neutron stars or black holes...

Galaxies, despite their huge masses just don't register. Proximity to massive objects is what counts.

[otseng]

No, in all three diagrams on the top row, the point denoted by the red dot experiences the same field in each position. The blue shell is mass, the white is void. If the dot moves closer to the shell the field (at the point) remains in balance because the greater part of the mass of the shell comes into "view" behind it (albeit at a distance). Maybe you're not seeing this because you're not familiar with the "magic" of Gauss's law which keeps the competing inverse-square law effects perfectly in balance. It's not meant to be an accurate depiction of what's going on, but this picture should help:



What I'm trying to show is that lots of weak vectors balance fewer strong ones. The sum of all vectors to the right of the point is always equal to those on the left (the same would apply if we also moved the point up or down). If I had more time I'd put signed numbers on the vectors and show them summing to zero, but you can probably imagine it for me

I understand your point about the red dot and experiencing greater gravitational force from the closer edge and thus having a net effect of zero. What I'm questioning is why would the white area remain the same even though the red dot is moving? Perhaps we are coming from two different angles here.

Let me try to explain my angle again.



Figure A would represent a bounded spherical universe. It is a homogenous universe with objects with the same mass uniformly distributed. Let's say each blue pixel is an object with the same mass.

Figure B has an observer (red dot) at the exact center of the circle. We can remove all the blue pixels with the red dot in the center of the circle. In this case, the entire circle would be yellow (just empty space). The net gravitational force on the observer would be zero.

In figure C, the red dot moves to the right. To simplify calculating the net gravitational force on the observer, we again remove all blue dots in a circle with the red dot in the center. The yellow circle would represent empty space. Then we'd have to calculate the sum of the gravitational forces from all the blue pixels with the red dot.

In figures D and E, the red dot continues to move to the right. And the cancelled out circle becomes smaller as it approaches the boundary.

In these figures, the yellow circle (cancelled out gravitational forces) changes as the red dot moves. The yellow circle would have a radius of the distance from the red dot to the closest boundary. And the red dot would be the center of the yellow circle.

[QED]

I understand your point about the red dot and experiencing greater gravitational force from the closer edge and thus having a net effect of zero. What I'm questioning is why would the white area remain the same even though the red dot is moving? Perhaps we are coming from two different angles here.

Well, if you do understand that the net gravitational effect on the red dot in the diagram cancels to zero, you should see that it makes no difference where (in the white space) the dot is. I.e. no matter where the red dot moves to in the white space, it will feel no greater or lesser attraction from the mass making-up the blue shell surrounding it.



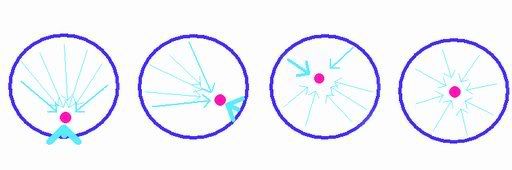
Above is a family of diagrams showing the red dot at various places and arrows that sum to zero (you intuitively know that it has zero net force when at the centre for example). I think it's the slightly counter-intuitive nature of this cancellation effect that's been throwing you off all along here.

Let me try to explain my angle again.

I think you'll find that you're not taking into account the distance squared fall-off of the field in your model.

[otseng]

Above is a family of diagrams showing the red dot at various places and arrows that sum to zero (you intuitively know that it has zero net force when at the centre for example). I think it's the slightly counter-intuitive nature of this cancellation effect that's been throwing you off all along here.

I think we're moving the same pieces back and forth on this.

Let's go back to your blue outer circle. What does that represent?

[QED]

Above is a family of diagrams showing the red dot at various places and arrows that sum to zero (you intuitively know that it has zero net force when at the centre for example). I think it's the slightly counter-intuitive nature of this cancellation effect that's been throwing you off all along here.

I think we're moving the same pieces back and forth on this.

Let's go back to your blue outer circle. What does that represent?

Here I've underlined where I had hoped to clarify it in my previous post:

no matter where the red dot moves to in the white space, it will feel no greater or lesser attraction from the mass making-up the blue shell surrounding it.

I started off the explanation with a model consisting of a point in empty space (the red dot on a white background) surrounded by a shell of mass (the blue blue circle) as a way of introducing the field cancellation effect. Intuitively we know (I would assume) that if all the mass in the universe was concentrated uniformly in such a shell, then at the exact center there would be no net force. Gauss's law then describes how (perhaps counter-intuitively) no matter where inside such a shell we look, there is still no net force (thanks to the Inverse Square law and spherical geometry -- if we consider this 2D representation to be slice through a 3D sphere)

This starting point allows us to ignore all homogeneous mass from our point out to the shell, as we can consider this region to be a continuous series of shells or, if you like, a thickening of the blue shell all the way to the point as shown in the lower three examples:



Now we can see that the only mass affecting our point is that which lies in the yellow region.

[otseng]

I started off the explanation with a model consisting of a point in empty space (the red dot on a white background) surrounded by a shell of mass (the blue blue circle) as a way of introducing the field cancellation effect. Intuitively we know (I would assume) that if all the mass in the universe was concentrated uniformly in such a shell, then at the exact center there would be no net force.

Here is where we diverge. I don't think the model would be an empty space surrounded by a shell. If we start off with being in the center of a solid sphere, all off the sphere would be cancelled out. There would not be any mass left after the cancellation effect.

[QED]

I started off the explanation with a model consisting of a point in empty space (the red dot on a white background) surrounded by a shell of mass (the blue blue circle) as a way of introducing the field cancellation effect. Intuitively we know (I would assume) that if all the mass in the universe was concentrated uniformly in such a shell, then at the exact center there would be no net force.

Here is where we diverge. I don't think the model would be an empty space surrounded by a shell.

What model are you referring to? I have presented a valid model for the purposes of assisting you with a thought-experiment that will show you what I could otherwise simply state and expect you to accept.

If we start off with being in the center of a solid sphere, all off the sphere would be cancelled out. There would not be any mass left after the cancellation effect.

Correct. That is the leftmost diagram on the bottom row. But if we start from here and refuse to consider the findings from the top row, we are likely to be led astray as you are. Please put your intuition on hold and look again at the point inside the hollow sphere...

Do you accept that if all the mass in the universe is uniformly concentrated in a spherical shell, then no points within the sphere experience any net attraction at all?